Tortuosity and microstructure effects in porous media: classical theories, empirical data and modern methods
This study provides a comprehensive review of tortuosity and its impact on the transport properties of porous media. It discusses the classical theories and equations related to tortuosity for flow, conduction, and diffusion. The study also highlights the evolution of these theories and their connection to methodologies such as tomography and 3D image analysis. In order to clarify the topic, a new classification scheme and nomenclature for different types of tortuosity are proposed. The study further examines empirical data on tortuosity-porosity relationships and identifies systematic patterns associated with different tortuosity types. It emphasizes the importance of clearly defining the tortuosity type and the underlying computation method. The study also reviews various methods for characterizing porous media and computing tortuosity, including 3D imaging, image processing, numerical simulation, stochastic geometry, and virtual materials testing. The study concludes by highlighting the advancements in methodology and the incorporation of additional relevant characteristics such as constrictivity and hydraulic radius, resulting in improved predictions of effective properties for complex microstructures.
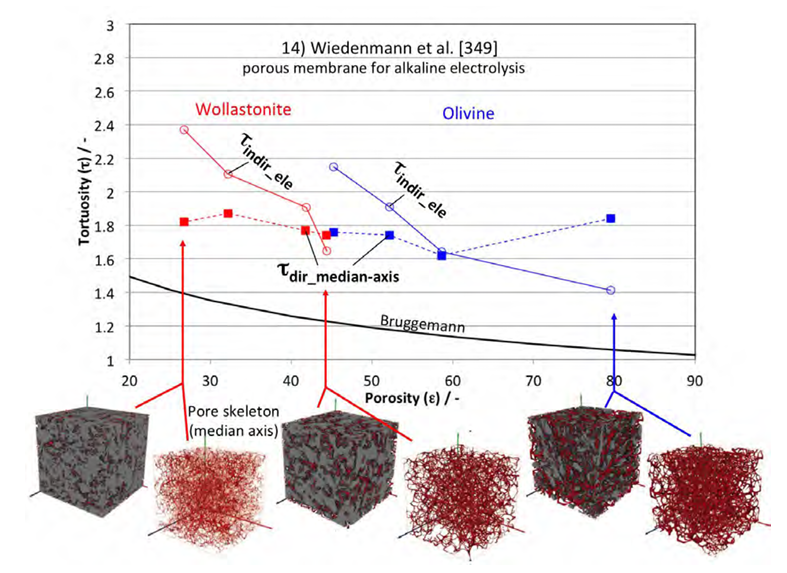
Comparison of indirect (τindir_ele_exp) vs. direct geometric tortuosities (τdir_medial_axis) for porous membranes consisting of wollastonite (red) and olivine (blue) from Wiedenmann et al. [349]. The geometric tortuosities (filled squares) vary in a narrow range (1.6-1.8), whereas the indirect tortuosities (open circles) show significant variation. The variation of effective properties and associated indirect tortuosities is attributed to variations of the size of characteristic bottlenecks and corresponding constrictivities.